More on Density and Games with Models, 5/13/20 Data
Somewhere out there on social media, I saw Sam Howard declaring that he would not respond to my argument about his earlier criticism of me. Whether he lacks the time or the integrity to acknowledge obvious errors and dishonest omissions on his part, I won’t guess.
Nonetheless, the question raised in our debate is an interesting one: Does it tell us anything useful to divide the number of cases of COVID-19 in a city by the population density? I say, “yes.” We know population density affects the spread of disease, and dividing one number by another gives us a ratio. If two cities have the same population density and one has more cases than the other, the ratios tell us that the reason for the difference is something other than density.
Of course, it doesn’t tell us what that other something is. If City A is just as dense and twice as large than City B, one would expect City A to have more cases. If City A is half as dense as City B but twice as large, and if density is a linear factor, then all things equal, they would have the same number of cases.
To stress, though, I wasn’t proposing to develop that formula. In my prior posts, I was only suggesting that a simple population density ratio suggested that density really could explain the fact that Central Falls has the most cases of COVID-19 per capita in Rhode Island without resorting to racism.
Maybe a different comparison that leaves open the possibility of race as an explanation would help progressives understand what I’m trying to say. Chelsea, Massachusetts, has 40,160 people, 67% of whom are Hispanic, and they live 15,903 people per square mile. Cambridge, Massachusetts, has 118,977 people, 9.2% of whom are Hispanic, and they live 16,470 people per square mile.
All things equal, one would expect Cambridge to have about three times as many cases of COVID-19 as Chelsea, but in fact, the ratio is close to reversed. Cambridge has 826 cases to Chelsea’s 2,244. Thus, the number of cases per population density for Cambridge is 0.05, versus 0.14 for Chelsea. So, population density doesn’t explain the difference.
Of course, if we wanted a formula that brought in all of the relevant factors, it would be more complicated. In some situations, we might try to adjust by size, as I did with my diagram of Central Falls and Pawtucket. Or where the population densities are pretty much the same, we might focus on the number of people. Alternately, if the disease reached one city earlier than another, we might want to take the amount of elapsed time into account.
In any event, if two different cities have the same population density and the same cases per density, that would suggest that density may be the decisive factor. To be sure, if Cambridge had Chelsea’s 0.14, the former would still have fewer cases per capita, because it has so many more people. Thus, the explanation for the difference is something other than total population or population density. It could be race, although I’d argue it’s more likely other factors that happen to be associated with a particular group at this point in time and location.
To go back to my original post on this topic, if we look at cases per population density for Central Falls, the number is way lower than looking at just population density would lead us to expect, which suggests that population density could explain the entire difference, with room to spare. Interestingly, if we add Central Falls to the Massachusetts comparison, the RI city has comparable population density. Yet, it has about 30% of the cases of Chelsea. Some of the difference is obviously explained by the fact that Central Falls has about half the total population, but not all of the difference. And since they have pretty much the same percentages of Hispanics, that variable offers no insight.
Again, I’m not coming to any positive conclusions from these numbers. I’m merely pointing out that those who wish to ascribe Central Falls’s per capita rate of cases to racism have more work to do.
Anyway… things continue to improve in Rhode Island overall. Here’s the daily chart of hospitalizations:
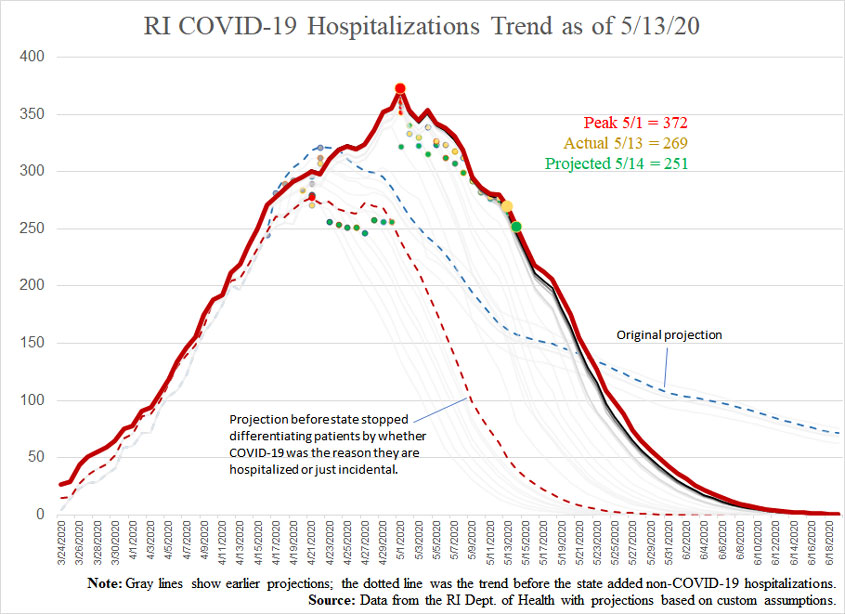
Projections versus actuals (date of reporting):
- Cases:
- Projection for 5/13: 11,755
- Actual for 5/13: 11,835
- Projection for 5/14: 11,972
- Hospitalizations:
- Projection for 5/13: 264
- Actual for 5/13: 269
- Projection for 5/14: 251
- Deaths:
- Projection for 5/13: 449
- Actual for 5/13: 462
- Projection for 5/14: